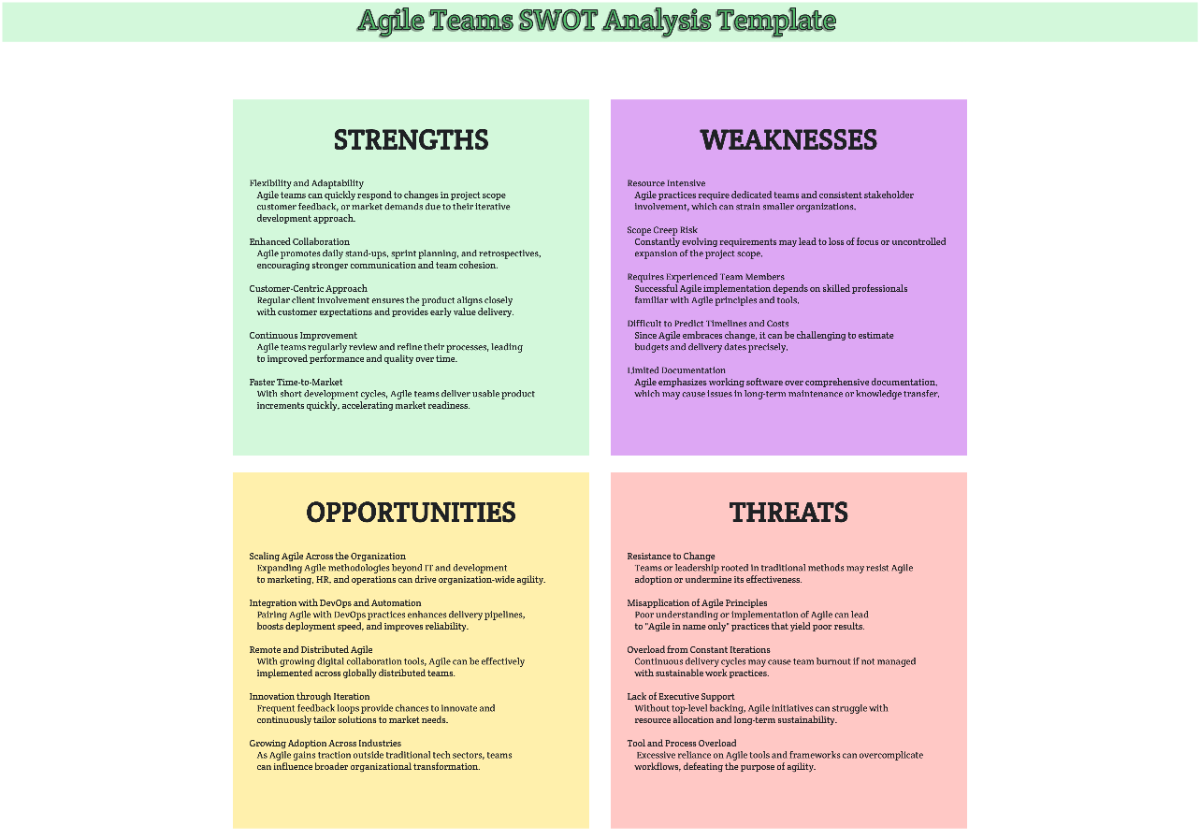
Real Analysis Syllabus

[Course Title]

Course Title	[COURSE TITLE]
Course Code	[COURSE CODE]
Office Hours	[OFFICE HOURS]
Class Location	[CLASS LOCATION]
Class Time	[CLASS TIME]
Class Duration	[DATE] - [DATE]

1. Course Description

This course is designed to introduce students to the foundational theories and concepts of Real Analysis. We will cover areas such as sequences, series, continuity, differentiability, integrability, topology of real numbers, and more. This is an advanced mathematics course suitable for students with a strong foundation in calculus.

2. Instructor Information

Instructor: [YOUR NAME]

Email: [YOUR EMAIL]

Organization: [YOUR COMPANY NAME]

3. Learning Objectives

Understand and apply fundamental concepts of real analysis.
Write clear and precise mathematical proofs.
Develop mathematical rigor and formal reasoning skills.
Recognize and solve problems in Real Analysis.
Understand how Real Analysis forms the backbone of many areas of mathematics.

4. Course Schedule

Week	Topic	Readings/Assignments
1	Foundation of Real Numbers	Chapter 1
2	Sequences and Series	Chapter 2
3	Continuity	Chapter 3
4	Differentiability	Chapter 4
5	Integrability	Chapter 5

5. Course Evaluation

As the semester comes to an end, an opportunity will arise for students to evaluate their course, encouraging them to provide feedback on the overall course, specific aspects such as teaching methods, course materials and their overall learning experience which will be crucial for further growth and improvement.

6. Required Reading and Materials

"Principles of Mathematical Analysis" by Walter Rudin
"Real and complex analysis" by W. Rudin
"Real Analysis: Modern Techniques and Their Applications" by Gerald B. Folland
Scientific calculator
Notebooks for note-taking and problem-solving exercises

7. Assignments and Assessments

Assignment	Due Date
Weekly problem sets to reinforce lecture topics.	[DUE DATE]
Two mid-term examinations to gauge student's understanding of the material.	[DUE DATE]
A comprehensive final examination covering all course material.	[DUE DATE]
Regular class participation, both in discussions and problem-solving.	[DUE DATE]
One research project a topic of the student's choice within real analysis.	[DUE DATE]

8. Examinations

Midterm Examination:
- The midterm examination will cover material from weeks 1 to 7.
- It will consist of both multiple-choice questions and proof-based questions.
Final Examination:
- The final examination will be comprehensive, covering all topics discussed throughout the semester.
- It will be held during the scheduled final exam period.

9. Course Policy

Attendance to all classes is mandatory.
Late submission of assignments will result in points deduction.
Academic integrity is paramount; any cases of plagiarism or cheating will result in severe penalties.
Students are expected to keep up with readings and assignments.
All questions or concerns regarding course content or requirements should be directed to the instructor without delay.

10. Grading Policy

A: 90-100%
B: 80-89%
C: 70-79%
D: 60-69%
F: Below 60%

Disclaimer

The syllabus for this course may be subjected to modifications or adjustments at any given time, as the course instructor sees fit and necessary for the overall learning process. Whenever any alterations in the syllabus are made, the instructor guarantees that it will be communicated promptly and accurately to each student to prevent any blurriness or misunderstanding around the matter.