Mathematics Class Notes
Prepared by: [Your Name]
I. Key Concepts
Understanding fundamental concepts is crucial for mastering mathematics. Below are some of the key concepts discussed in the class:
Algebraic Expressions
Functions and Graphs
Calculus: Differentiation and Integration
Geometry and Trigonometry
Probability and Statistics
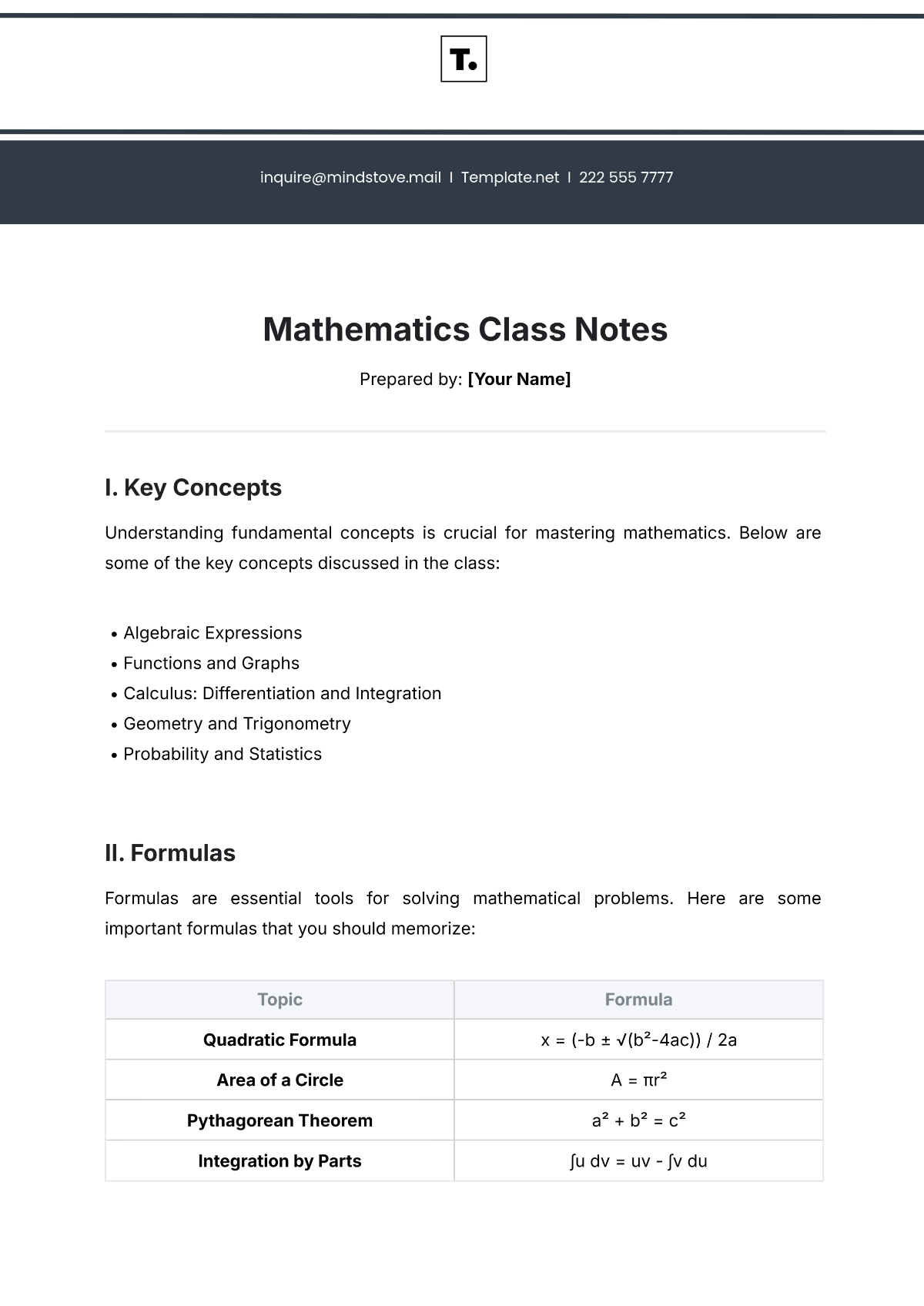
II. Formulas
Formulas are essential tools for solving mathematical problems. Here are some important formulas that you should memorize:
Topic | Formula |
|---|---|
Quadratic Formula | x = (-b ± √(b²-4ac)) / 2a |
Area of a Circle | A = πr² |
Pythagorean Theorem | a² + b² = c² |
Integration by Parts | ∫u dv = uv - ∫v du |
III. Theorems
Theorems provide a formal statement of a mathematical principle. Here are some significant theorems covered in class:
Pythagorean Theorem: In a right-angled triangle, the square of the hypotenuse is equal to the sum of the squares of the other two sides.
Fundamental Theorem of Calculus: Connects differentiation and integration, providing an efficient method for evaluating definite integrals.
Intermediate Value Theorem: If a continuous function, f(x), has values of opposite sign inside an interval, then it must have a root in that interval.
IV. Problem-Solving Techniques
Problem-solving is a critical skill in mathematics. Here are some techniques discussed in class:
Understanding the problem
Devising a plan
Carrying out the plan
Reviewing and extending
V. Examples
Applying concepts to solve problems reinforces understanding. Consider the following examples:
Example 1: Solving a Quadratic Equation
Given the quadratic equation x² - 5x + 6 = 0, solve for x.
Solution: Apply the quadratic formula:
x = [5 ± √(5²-4*1*6)] / 2*1 = [5 ± √(1)] / 2 = (6,1)
Example 2: Calculating the Area of a Circle
Find the area of a circle with a radius of 4 cm.
Solution: Use the area formula A = πr²:
A = π(4)² = 16π cm²